Whenever I write and talk about education I have always been cautious about using the word "teaching" without appending the word "learning" - normally describing it as "teaching/learning". I can trace this caution to my teacher training days, when the dominant paradigm was based on Piaget's ideas of development.
A teacher does not teach but causes learning to occur.
Of course, I knew that I was teaching (and students might be learning), and yet my training caused me to minimise the direct teaching aspect.
Subsequently, reading Bruner, Vygotsky and Friere, I developed a more complete explanation. But still, I have always considered the learner as paramount - what the teacher does (by itself) is insufficient.
Wood (p75, 1988) considers effective instruction and in particular describes how an "expert's" knowledge endows them with the ability to perceive organisation and structure, whereas a "novice's" perception is piecemeal and fragmented. Teaching can be described as changing students from novices towards being experts, but this definition does not describe the process that achieves it.
Expertise "cannot be reduced to an aggregate of elementary skills acquired stepwise at inferior levels" (Dreyfus and Dreyfus, 1986), since experts tend to react globally, by "intuition on the basis of rich experience". Fischbein, in 1987, argued that thinking itself would be impossible if we could not rely on immediate, self-evident intuitions (Nesher and Kilpatrick, 1990), and promoted an intuitionistic approach to mathematical education (my particular interest area). However, whereas providing insights into how "experts" work is a useful experience for students, copying the seemingly effortless expert approach will not turn novices into experts.
Teaching mathematics should not be solely about progressing through a hierarchical set of skills, nor taught through definitions and axioms. Such a formal hierarchy and structure develops FROM the learning experience rather than BEING the learning experience.
Glaserfeld's view, that the learner constructs her own meanings, is the more useful approach. His definition of learning (1989) is somewhat generalised ("to have drawn conclusions from experience and acted accordingly"), but his assertion that knowledge is not a transferable commodity nor that communication ensures the conveyance of it is surely a good starting point.
I know that this is not trendy - connectivism being the MOOC #change11 approach (and one which I am following) - but at school level constructivism is far more useful.
Students (in schools), then, should construct their own knowledge. The teaching and activity sequence is largely determined by the teacher. Activities are selected for them that enable them to "construct local expertise" by using the contingent teaching approach (described by Wood, p80, 1988).
Is "telling" teaching?
It isn't if you believe that a learner constructs their own knowledge. The approach that one follows as a teacher rests, crucially, on the way teaching and learning is modelled in the teacher's (and learner's) mind.
Bruner (1986), with his consideration of the part language plays and Glaserfeld's (1989) view of knowledge, form the substance to make sense of Friere's (1989) initially difficult but illuminating approach.
Bruner point to the way language can be used "to express stance and counter stance (...) leav(ing) place for reflection, for meta-cognition" (p129). What he implies is that language is fundamental to the learning process since it is used for communication and refinement of ideas, and reflection and refinement of ideas. He states that it is the shared use of language "which unlocks others minds to us". I am not convinced that using language necessarily leads to a better and more accessible storing of ideas and information by the learner, but the repeated rehearsing and altering of ideas clearly brings about quality learning.
Glaserfeld's view of knowledge can be summarised as follows:
- knowledge is not a physically exact representation of the environment but a "mapping of personally viable ways" of achieving goals;
- knowledge is constructed by the individual and NOT conveyed or instilled by diligent perception or linguistic communication;
- language is used not as means of transmission but as a means of communicating, which allows the teacher to constrain and guide. (sg p58)
With regards to the second view, I agree with his contention that knowledge is constructed by the individual but I cannot agree that knowledge
cannot be conveyed by linguistic communication. Clearly such linguistic communication, whether oral to aural or visual (presentations, resources, etc), is used as one of the means to convey knowledge in classrooms everywhere. That this is not sufficient to ensure learning, I agree with.
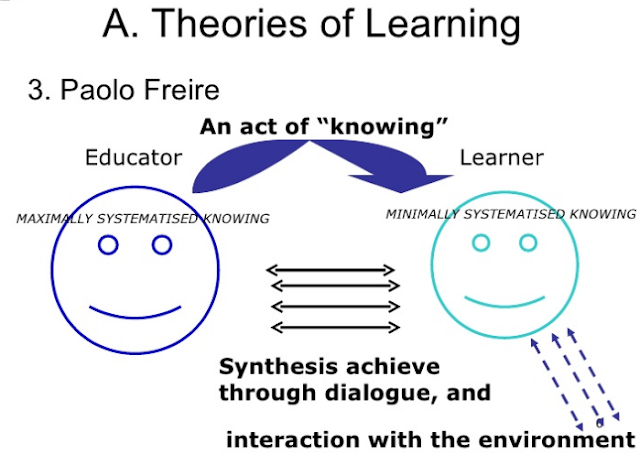
His third view of knowledge, dealing with the use of language by the teacher to constrain and guide, is a useful starting point to how Friere (1989) considers learning. He defines learning as an act of knowing which takes place through a process of action, reflection upon action, and new action. This is my version of Friere's description of education:
He describes the educator's role as being that of helping the learner to criticise her view of reality, and re-adjust it. This seems to me to be a better model to follow since it focuses on the educator/learner interaction, does not get bogged down in descriptive dichotomies, and does not task the learner alone with determining the viability of her knowledge. I repeat, I am writing about what goes on in schools daily, not higher education and MOOCs.
Why this journey into traditional educational theory? Well, in looking at the place of technology in all this, and the opportunities it might give us, I have gone back to the original pre-technology pedagogy. Is it still sound? Does it still apply?
References:
Dreyfus, HL and Dreyfus, SE (1986) in Nesher, P and Kilpatrick J [eds] (1990)
Mathematics and Cognition - a research synthesis by the International Group for the Psychology of Mathematical Education; Cambridge University Press.
Freire, P (1989)
The Politics of Education in Murphy, P and Moon, B (eds): Developments in Learning and Assessment, Hodder and Stoughton, and the Open University, London.
Glaserfeld, E von (1989)
Learning as a Constructivist Activity in Murphy, P and Moon, B (eds): Developments in Learning and Assessment, Hodder and Stoughton, and the Open University, London.
Nesher, P and Kilpatrick, J (eds) (1990)
Mathematics and Cognition - a research synthesis by the International Group for the Psychology of Mathematical Education; Cambridge University Press.
Wood, D (1988)
How Children Think and Learn, Blackwell, Oxford.